Thursday, October 17, 2024

Thermosteric Sealevel Change Revisited - 4

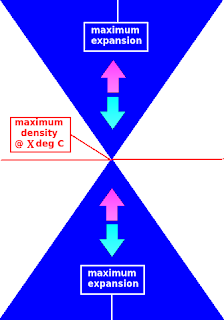
Fig. 1  -3 C is 26.6 F

In this post the blanks are filled in with the relevant temperatures that take the perplexing reality out of thermal expansion and contraction.

The graphics I have used in this series show that it is no longer a mystery.

It is a scientific fact that the maximum density temperature is also a border line that activates an eye catching transition in seawater.

But more than that the not-often-discussed border line is instructive for those who would try to figure out the dynamics of temperature change induced volume change (thermal expansion and contraction) in seawater they are studying.

To make it easier to comprehend two HTML tables are provided below to show the mysterious event in two temperature paths; one from warm to cold and the other from cold to warm.

The only change in the relevant operative variables is the in situ temperature (T) which begins at +19 deg. C and ends at -10 deg. C in one experiment (Fig. 1), but is reversed (begins at -10 deg. C and ends at +19 deg. C) in the other experiment (Fig. 2).

Here are the two HTML tables:

Table 1

The HTML table below details the results of a C++ test program which calculates volume and density as the Conservative Temperature (CT) changes. The constant values are: depth (30m), lat (30 N), lon (10 W), SP (salinity 32). T (in situ temperature) varies. The bold line is maximum density.

'Row' means test's iteration position

'CT' means the TEOS-10 Conservative Temperature

'T' means the in situ seawater temperature

'Density' means the TEOS-10 density value

'Volume' means the TEOS-10 calculated amount of ocean space the seawater column fills.

Row CT T density volume
1 19.0767 19 1022.87 9.77644 x 10-4
2 18.0723 18 1023.11 9.77408 x 10-4
3 17.068 17 1023.35 9.77181 x 10-4
4 16.0638 16 1023.58 9.76963 x 10-4
5 15.0597 15 1023.8 9.76754 x 10-4
6 14.0558 14 1024.01 9.76554 x 10-4
7 13.052 13 1024.21 9.76363 x 10-4
8 12.0484 12 1024.4 9.76181 x 10-4
9 11.0448 11 1024.58 9.76009 x 10-4
10 10.0414 10 1024.75 9.75847 x 10-4
11 9.03806 9 1024.91 9.75695 x 10-4
12 8.03483 8 1025.06 9.75554 x 10-4
13 7.0317 7 1025.2 9.75422 x 10-4
14 6.02866 6 1025.32 9.75302 x 10-4
15 5.02568 5 1025.44 9.75192 x 10-4
16 4.02276 4 1025.54 9.75093 x 10-4
17 3.01988 3 1025.63 9.75007 x 10-4
18 2.01702 2 1025.71 9.74931 x 10-4
19 1.01416 1 1025.78 9.74869 x 10-4
20 0.0112749 0 1025.83 9.74818 x 10-4
21 -0.991654 -1 1025.87 9.74781 x 10-4
22 -1.99466 -2 1025.9 9.74757 x 10-4
23 -2.99777 -3 1025.91 9.74747 x 10-4
24 -4.00103 -4 1025.9 9.74751 x 10-4
25 -5.00446 -5 1025.88 9.7477 x 10-4
26 -6.00812 -6 1025.85 9.74804 x 10-4
27 -7.01203 -7 1025.79 9.74855 x 10-4
28 -8.01627 -8 1025.72 9.74921 x 10-4
29 -9.02086 -9 1025.64 9.75005 x 10-4
30 -10.0259 -10 1025.53 9.75107 x 10-4

Fig. 2  -3 C is 26.6 F

Table 2

The HTML table below details the results of a C++ test program which calculates volume and density as the Conservative Temperature (CT) changes. The constant values are: depth (30m), lat (30 N), lon (10 W), SP (salinity 32). The "T" (in situ temperature) varies. The bold line is maximum density.

'Row' means test's iteration position

'CT' means the TEOS-10 Conservative Temperature

'T' means the in situ seawater temperature

'Density' means the TEOS-10 density value

'Volume' means the TEOS-10 calculated amount of ocean space the seawater column fills.

Row CT T density volume
1 -10.0259 -10 1025.53 9.75107 x 10-4
2 -9.02086 -9 1025.64 9.75005 x 10-4
3 -8.01627 -8 1025.72 9.74921 x 10-4
4 -7.01203 -7 1025.79 9.74855 x 10-4
5 -6.00812 -6 1025.85 9.74804 x 10-4
6 -5.00446 -5 1025.88 9.7477 x 10-4
7 -4.00103 -4 1025.9 9.74751 x 10-4
8 -2.99777 -3 1025.91 9.74747 x 10-4
9 -1.99466 -2 1025.9 9.74757 x 10-4
10 -0.991654 -1 1025.87 9.74781 x 10-4
11 0.0112749 0 1025.83 9.74818 x 10-4
12 1.01416 1 1025.78 9.74869 x 10-4
13 2.01702 2 1025.71 9.74931 x 10-4
14 3.01988 3 1025.63 9.75007 x 10-4
15 4.02276 4 1025.54 9.75093 x 10-4
16 5.02568 5 1025.44 9.75192 x 10-4
17 6.02866 6 1025.32 9.75302 x 10-4
18 7.0317 7 1025.2 9.75422 x 10-4
19 8.03483 8 1025.06 9.75554 x 10-4
20 9.03806 9 1024.91 9.75695 x 10-4
21 10.0414 10 1024.75 9.75847 x 10-4
22 11.0448 11 1024.58 9.76009 x 10-4
23 12.0484 12 1024.4 9.76181 x 10-4
24 13.052 13 1024.21 9.76363 x 10-4
25 14.0558 14 1024.01 9.76554 x 10-4
26 15.0597 15 1023.8 9.76754 x 10-4
27 16.0638 16 1023.58 9.76963 x 10-4
28 17.068 17 1023.35 9.77181 x 10-4
29 18.0723 18 1023.11 9.77408 x 10-4
30 19.0767 19 1022.87 9.77644 x 10-4

The numbers in the HTML tables are generated by the free TEOS-10 C++ software library.

I only furnish the constant values and the beginning "T" (in situ temperature) which is iterated by the C++ program that is using the TEOS-10 library functionality.

In other words the event horizon for the maximum density temperature is a result or discovery of the Gibbs function "built into" the TEOS-10 library.

As has been indicated previously on Dredd Blog, Gibbs was the correct choice to consider when TEOS-10 was developed:

"Listening to Gibbs, who is perhaps the most influential historical voice in ocean thermodynamics (encapsulated in TEOS-10) would also help:
'Albert Einstein called him 'the greatest mind in American history.' Gibbs’s studies of thermodynamics and discoveries in statistical mechanics paved the way for many of Einstein’s later discoveries.'
(American Physical Society [they "lost" the link so, it's off to the Wayback Machine ... which is under a "denial of service attack"] or APSloane). Especially since 'encapsulated' means:
'TEOS-10 is based on a Gibbs function formulation from which all thermodynamic properties of seawater (density, enthalpy, entropy sound speed, etc.) can be derived in a thermodynamically consistent manner.'

(In Search Of Ocean Heat - 5). Thus, the Dredd Blog assertion that whether seawater will increase in volume (thermal expansion) or decrease in volume (thermal contraction) depends on the temperature of the seawater at the time the temperature changes.

On to the next subject.

The previous post in this series is here.

Ode To The Warming Commentariat:

Posted by at 1 comment:

Wednesday, October 16, 2024

Thermosteric Sealevel Change Revisited - 3

Fig. 1 Is it Verifiable or Falsifiable?

I. Background

There is so much disbelief and lack of analysis in the realm of discerning "thermal expansion" on the part of the Warming Commentariat and some oceanographers as well, that once again I must review one of the Dredd Blog assertions about it.

The issue I am focusing on today is my assertion over the years that thermal expansion does not take place in all cases where the seawater temperature increases.

In fact I have gone so far as to say that it depends on the temperature of the seawater at the time the temperature changes (ergo in some cases the increase of the seawater temperature will cause thermal contraction meaning the seawater will "shrink" which is to say decrease in volume and increase in density.

Water is one of the only things where this is the case, which means that the hypothesis seems to be a mythical rather than a scientific concept.

In fact this past week I have doubted myself to the point of writing a C++ program that points out that the hypothesis can be verified or falsified, which at least makes it a valid hypothesis (Falsifiability, Popper; Verifiability).

II. The Brass Tacks

As I indicated above "getting down to the brass tacks" in this case began with the writing of a C++ program.

The exercise was to show that if the temperature of seawater warms, the result in terms of volume increase  or to the contrary volume decrease DEPENDS ON THE TEMPERATURE OF THE WATER AT THE TIME the temperature change takes place. 

If the temperature is above the temperature of maximum density (see Fig. 1) then there will be thermal expansion, but to the contrary if the temperature is below the temperature of maximum density, there will be thermal contraction.

III. The Experimental Data

Today's appendix (APNDX TSCR 3) contains an HTML table and a graph which together show the results of the following process.

1) these initial variables are required:

--------------------------------
in situ variables
--------------------------------
SP = in situ salinity
T = in situ temperature (deg. C)
depth = in situ depth (meters)
lat = latitude
lon = longitude

2) with those in situ variables, use TEOS-10 software to calculate TEOS-10 variables:

--------------------------------
TEOS-10 variables
--------------------------------
Z = geodesic height (negative)
P = pressure
SA = Absolute Salinity
CT = Conservative Temperature
TEC = thermal expansion coefficient
3) by using the following TEOS-10 functionality:
------------------------------------------
TEOS-10 methods/functions
------------------------------------------
(Z)
gsw_z_from_depth(depth);

(P)
gsw_p_from_z(Z, lat,0.0,0.0);

(SA)
gsw_sa_from_sp(SP, P, lon, lat);

(CT)
gsw_ct_from_t(SA, T, P);

(specific volume)
gsw_specvol(SA,CT,P);

(Density)
>gsw_rho(SA,CT,P);

(TEC)
gsw_alpha(SA, CT, P);

The appendix contains displays of the results which seem to confirm the hypothesis (APNDX TSCR 3).

IV. Closing Comments

If I pour water onto its forms (steam, liquid, ice), for instance ice, it will shrink in volume as it transitions to water.

Same with steam.

This is not an easy-to-grasp situation, so I will keep working on it until I find a better way to falsify it or verify it.

Helpful suggestions accepted.

The next post in this series is here, the previous post in this series is here.

Posted by at No comments:

APNDX TSCR 3

This is an appendix to: Thermosteric Sealevel Change Revisited - 3


Example 1

 

Example 2:

The HTML table below details the results of a C++ test program which calculates volume and density as the Conservative Temperature (CT) changes. In rows 1-20 there is a decreasing CT value, with row 14 reaching maximum density and Row 20 reaching lowest CT value. Rows 21-30 have an increasing CT value, with row 26 reaching maximum density again.

'Row' means test's iteration position

'CT' means the TEOS-10 Conservative Temperature

'T' means the in situ seawater temperature

'Density' means the TEOS-10 density value

'Volume' means the TEOS-10 calculated amount of ocean space the seawater column fills.

Row CT T density volume
1 10.0414 10 1024.75 9.75847 x 10-4
2 9.03806 9 1024.91 9.75695 x 10-4
3 8.03483 8 1025.06 9.75554 x 10-4
4 7.0317 7 1025.2 9.75422 x 10-4
5 6.02866 6 1025.32 9.75302 x 10-4
6 5.02568 5 1025.44 9.75192 x 10-4
7 4.02276 4 1025.54 9.75093 x 10-4
8 3.01988 3 1025.63 9.75007 x 10-4
9 2.01702 2 1025.71 9.74931 x 10-4
10 1.01416 1 1025.78 9.74869 x 10-4
11 0.0112749 0 1025.83 9.74818 x 10-4
12 -0.991654 -1 1025.87 9.74781 x 10-4
13 -1.99466 -2 1025.9 9.74757 x 10-4
14 -2.99777 -3 1025.91 9.74747 x 10-4
15 -4.00103 -4 1025.9 9.74751 x 10-4
16 -5.00446 -5 1025.88 9.7477 x 10-4
17 -6.00812 -6 1025.85 9.74804 x 10-4
18 -7.01203 -7 1025.79 9.74855 x 10-4
19 -8.01627 -8 1025.72 9.74921 x 10-4
20 -9.02086 -9 1025.64 9.75005 x 10-4
21 -8.01627 -8 1025.72 9.74921 x 10-4
22 -7.01203 -7 1025.79 9.74855 x 10-4
23 -6.00812 -6 1025.85 9.74804 x 10-4
24 -5.00446 -5 1025.88 9.7477 x 10-4
25 -4.00103 -4 1025.9 9.74751 x 10-4
26 -2.99777 -3 1025.91 9.74747 x 10-4
27 -1.99466 -2 1025.9 9.74757 x 10-4
28 -0.991654 -1 1025.87 9.74781 x 10-4
29 0.0112749 0 1025.83 9.74818 x 10-4
30 1.01416 1 1025.78 9.74869 x 10-4
Posted by at No comments:
Subscribe to: Posts (Atom)